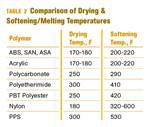
PART 2 Heat Deflection Temperature vs. Dynamic Mechanical Analysis

Several years ago, I was working with a client on a part being molded from an unfilled PBT polyester. The part was exhibiting excessive deformation at the operating temperature of 140 C (284 F). The engineers I was working with were baffled by the failures. They had a data sheet that showed that the material had a heat-deflection temperature (HDT) of 153 C (309 F). Consequently, in their minds, this was a guarantee of success at 140 C. This reflects a lack of understanding regarding the meaning of HDT. What is actually being measured?

This question was addressed, at least in part, 40 years ago in a paper written by Michael Takemori and presented at the Society of Plastics Engineers’ ANTEC. He showed that the HDT was a measurement of the temperature at which the modulus of a material declined to a particular value due to an increase in temperature. The exact modulus value will depend upon the geometry of the specimen. However, a number of different calculations using these varied geometries show that the HDT at 66 psi (0.455 MPa) is associated with a modulus between 27 and 35 ksi (190-240 MPa), which is the modulus at room temperature of an LDPE with a density in the range of 0.916-0.919 g/cm³. The HDT at 264 psi, a stress four times higher, will be associated with a modulus four times higher, or in the vicinity of 108-140 ksi (750-960 MPa), the modulus of an HDPE on the lower end of the density spectrum (0.946-0.948 g/cm³).

Here is a quote from the introduction to Takemori’s paper. “Since this measured HDT corresponds to a single point on the deflection-temperature curve, a great deal of potentially useful information is discarded when one merely reports the heat-distortion temperature.” Takemori provided some examples of these curves he referred to in order to illustrate the position of the HDT value on the curve. It is this discarded information that gets so many designers and engineers in trouble when they rely on a value that expresses a single point on a very useful curve, a curve that is provided by dynamic mechanical analysis (DMA).

The HDT on the data sheet for the unfilled PBT in question had been measured at 66 psi. When we located a more complete data sheet, it showed an HDT at 264 psi of 53 C (129 F), a full 100^o C lower than the value my client was counting on as a reliable indicator of behavior under load. They were stunned by this drastic reduction in the measured HDT value. What could possibly explain such a large change? Worse yet, a close inspection of the fine print on the data sheet showed that the test specimens used to generate the test results were 6.4 mm (0.252 in.) thick.

The part that was failing had a nominal wall thickness of 2.5 mm (0.100 in). There was one more important difference between the application environment and the HDT test. The application temperature of 140 C was often maintained for eight to 10 hr at a time while the part was under load. Consequently, some provisions were needed to account for creep. The HDT test involves heating the test specimen at a constant rate of 2^o C/min until the required deflection is achieved. Once that point is reached, the test stops. It is true that because the HDT test utilizes a constant load, creep is a component of the test. But the test duration in this case is barely over 1 hr. And the effects attributable to creep are conflated with those related to the rising temperature in a way that is very unclear unless additional data is available about the temperature-dependent behavior of the material.

The accompanying graph shows the curve provided by DMA for the PBT that was failing in my client’s application. This shows the full property spectrum for the material as a function of temperature. The first revelation for the prospective user of this material is the rapid change in modulus that occurs between 45 C and 100 C. Over this relatively narrow temperature range the modulus of the material decreases by over 80%. By the time the temperature reaches that of the application environment of interest, the material retains only 11% of the stiffness it possesses at room temperature.

This readily explained the failures my client was experiencing. This rapid change in modulus is associated with a very important phenomenon that all polymers exhibit, called the glass transition. This transition is a relaxation that involves the amorphous portion of the material. Once the material has passed through this transition, only the crystals contribute to the load-bearing capability of the material. In most unfilled semi-crystalline materials, an associated decline in modulus of 80-90% is typical. And in most of these polymers the HDT measured at 66 psi is located on this lower plateau. Temperatures in this region are relatively high, giving the impression that the material is structurally capable at these elevated temperatures.

The glass transition, and the rapid and significant decline in modulus that it produces, is also behind the large difference between the HDT values at 66 psi and 264 psi. The higher modulus associated with the HDT measured at 264 psi takes us into the glass-transition region, where each incremental change in temperature produces a much larger change in modulus than that which occurs when we are operating above or below the glass transition. Many unfilled semi-crystalline polymers, including PBT, the various nylon polymers, PPA, PPS, and PEEK will exhibit these large temperature differences between the two HDT values simply because the associated modulus value for the lower stress level typically occurs on the lower plateau where only the crystal structure governs performance while the modulus for the higher stress level frequently appears somewhere in the glass transition region where the modulus is in rapid decline.

The DMA curve provides several obvious advantages to the HDT numbers. First, it provides a complete and quantitative picture of the effect that temperature has on the load-bearing properties of a material. Anyone serious about designing a product and selecting an appropriate material should be able to understand the benefit of having a complete modulus-temperature curve instead of relying on what amounts to one or two points on this curve. Finite-element analysis (FEA), a tool for predicting performance at operating conditions, relies at minimum on an accurate input value for modulus. In most cases, the value that is used is the one on the data sheet, which is measured at 23 C. With the DMA curve, the exact modulus at the application temperature can be used.

Second, the ability to observe the effect of changing temperature on modulus allows the design engineer to identify temperature regions where the material may be susceptible to more significant levels of creep or stress relaxation. One of the principles of viscoelastic behavior is that increasing temperature and increasing time have the same effect on load-bearing properties. In a formal treatment of viscoelasticity this principle often gets lost in the complexity of the math. But it can be understood more simply by observing the changing slope of the modulus curve in the graph. Above and below the glass transition, each temperature increase of 5^o C reduces the modulus by about 1700-3500 psi (12-24 MPa). This equates to a condition where an applied stress will result in a relatively small amount of time-dependent movement. Within the steepest portion of the glass transition region (50-75 C), this same 5^o C change produces modulus reductions of 30,000-45,000 psi (210-310 MPa). A part produced in this material and placed under load in this temperature range will deform substantially in relatively short period of time.

Third, because the heating rate of the DMA test can be controlled to be identical to that of the HDT test (2^o C/min), the amount of time required to generate these complete curves is essentially the same as the time needed to determine the HDT.

The question then is, why are we content with using HDT values, when the technology for generating these complete curves has been around for over 30 years? And why are so many practitioners in the field of polymers either unaware of this option or insistent upon defending the use of obsolete techniques to characterize elevated-temperature performance? This is a question we will take up in our next installment.

ABOUT THE AUTHOR: Mike Sepe is an independent, global materials and processing consultant whose company, Michael P. Sepe, LLC, is based in Sedona, Ariz. He has more than 40 years of experience in the plastics industry and assists clients with material selection, designing for manufacturability, process optimization, troubleshooting, and failure analysis. Contact: (928) 203-0408 • mike@thematerialanalyst.com.