The Effects of Stress on Polymers
Previously we have discussed the effects of temperature and time on the long-term behavior of polymers. Now let's take a look at stress.
In the last two columns, we have discussed the effects of temperature and the effects of time on the long-term behavior of polymers, and we took a small side trip while covering the subject of time to treat the topic of strain rate.
The third axis that completes the picture is stress. Normally we think of stress as a short-term property since data sheets provide information only at selected points on the stress-strain curve. These points usually involve a catastrophic event such as the point where the material yields or completely fails. However, this only tells us what we should not do with a material; it provides no guidance on what we can expect in a successful application.
Relationship of Stress to Strain
Assuming that the stress-strain curve is generated at the application temperature, the yield or failure point will provide a useful upper limit as long as the duration of the application is very short. However, as the time frame of the loading increases, we find that in polymer systems the allowable stress that can be applied must be reduced if the part is to function as expected for the life of the product.
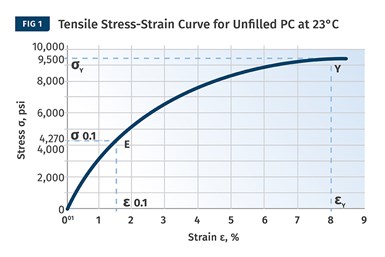
FIG 1 A stress-strain curve at room temperature for an unfilled PC shows that the relationship of stress to strain is linear for only a small portion of the curve. The modulus of the material, which is the ratio of stress to strain, is calculated in this linear region. For most polymers the upper limit for this linear region occurs at or below 1% strain.
Figure 1 provides a stress-strain curve generated at room temperature for an unfilled polycarbonate. It shows a yield stress of 9500 psi (65.5 MPa) and a yield strain of 8%. We already know that the shape of this curve will change as a function of temperature, but for now let’s assume that the application environment will always remain at room temperature and these values will serve as a good guideline for the capabilities of the material.
If we examine this curve closely we can see that the relationship of stress to strain is linear for only a small portion of the curve. The modulus of the material, which is the ratio of stress to strain, is calculated in this linear region. For most polymeric materials the upper limit for this linear region occurs at or below 1% strain. For many materials this proportional limit takes place even below 0.5% strain. Beyond this point ever smaller increments of stress are required to produce the next interval of strain.
For example, since the modulus of PC is typically about 340,000 psi (2340 MPa), a strain of 0.5% is associated with a stress of 1700 psi (11.7 MPa). If the behavior of the material were strictly linear, then it would require three times this stress, or 5100 psi (35.1 MPa) to attain a strain of 1.5%. However, the graph shows that the stress required to reach 1.5% strain is only 4270 psi (29.45 MPa), or about 84% of what would have been required if the material maintained a proportional stress-strain relationship. It is apparent that the slope of the curve has started to decrease at this 1.5% strain and further increases in stress produce a continued decrease in this slope as the curve approaches the yield point.
This has implications for long-term behavior under load. We already know that a polymer system under constant stress exhibits a continual increase in deformation known as creep strain. The rate at which creep strain increases is related to the slope of the stress-strain curve at the applied stress.
For example, if a stress of 2000 psi (13.8 MPa) is applied to a design element produced from this PC, it will exhibit an instantaneous strain of approximately 0.6%. Experiments have shown that if this stress is maintained for 1000 hr this strain will increase to 0.8%, about one-third greater than the initial strain.
However, if we elevate the initial strain by a factor of 2.5 to 5000 psi (34.5 MPa), the initial strain increases by a factor of not 2.5 but more than three, to 1.9%, because we are now well into the non-linear or plastic deformation portion of the curve. And if this stress is maintained for the same 1000-hr period, the strain grows to 3%, a much faster rate of increase than we saw at the lower stress level.
Higher stresses give rise to faster increases in creep strain. Since all materials have a limiting strain beyond which they cannot function, this faster increase in strain means that the product life will shorten with increasing stress. Said another way, the longer a product must perform in the field, the lower the initial stress must be on the material to ensure proper functioning of the product.
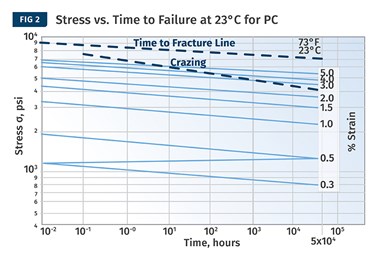
FIG 2 Higher stresses give rise to faster increases in creep strain. The longer a product must perform in the field, the lower the initial stress must be on the material to ensure proper functioning of the product. The “Time to Fracture” line gives the relationship between stress and time to failure at room temperature. The line marked “crazing” represents the relationship between the applied stress and the time required to initiate crazing
This principle can be observed in Fig. 2. This plot contains a great deal of information about the long-term performance of the PC. However, one of the data points of greatest interest to this discussion is the “Time to Fracture” line. This gives the relationship between stress and time to failure at room temperature. Note that at very short time frames of less than an hour the maximum allowable stress is nearly equivalent to the yield stress measured in Fig. 1.
However, as the time scale increases, the allowable stress declines. In approximately 50 hr the maximum allowable stress has declined by almost 16% to 8000 psi (55.2 MPa) and by the time we reach the 50,000-hr point (about 5.7 yr) the value has declined to 7000 psi (48.3 MPa).
This is an important consideration that shows why knowing the expected lifetime of a product is important to gauging the mechanical capabilities of the materials selected for the application. One of the key causes of failure involves extending the warranty of a product without taking the time to reassess the long-term capability of the materials of construction. While the behavior of metal components may be essentially constant when time, stress, and temperature are considered, this is not the case for plastic compounds.
‘Crazing’
There is another line on the plot in Fig. 2 that is of interest. It is the line marked “Crazing.” This represents the relationship between the applied stress and the time required to initiate crazing. Crazing represents the initial stages of failure in a material, particularly in amorphous polymer like PC. If crazing occurs in the presence of certain chemicals that can act as stress-cracking agents, crazing leads quickly to outright failure.
Notice that the stresses required to produce crazing are lower than those required to produce fracture and that the slope of this line is more severe than the “Time to Fracture” line. The stress required to produce failure in 50,000 hr produces crazing in less than 1 hr. At 50,000 hr, crazing will occur at a stress level of 4000 psi (27.6 MPa), less than 60% of the stress required to produce fracture when no chemicals are present.
Stress-cracking agents are everywhere. They can be in cleaning agents, lubricants and greases, plasticizers in mating rubber components, and protective coatings on metal parts that may be assembled into molded bosses. Stress cracking is the most common cause of plastic product failure and is particularly difficult to manage since the time frame of this type of failure is typically weeks or months. Therefore, the problem does not show up until a significant amount of product has been shipped.
This concludes our discussion on the key factors that influence the long-term performance of a plastic material. To recap, they are temperature, time, and stress. And they are interrelated. Anytime one of these three parameters changes, the other two must also be adjusted in order to ensure proper product function. An increase in temperature will require a corresponding decrease in some combination of product lifetime and applied stress.
Similarly, extending the lifetime expectations of a product will require a reduction in applied stress, temperature, or some combination of the two. This interaction, along with the shortage of good data that captures this behavior, is part of the challenge of selecting the appropriate polymeric material for an application.
Related Content
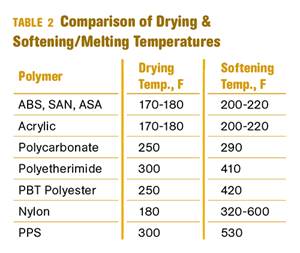
Why (and What) You Need to Dry
Other than polyolefins, almost every other polymer exhibits some level of polarity and therefore can absorb a certain amount of moisture from the atmosphere. Here’s a look at some of these materials, and what needs to be done to dry them.
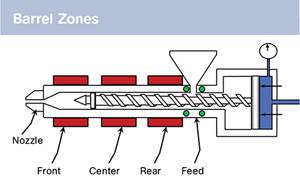
Read MoreHow to Set Barrel Zone Temps in Injection Molding
Start by picking a target melt temperature, and double-check data sheets for the resin supplier’s recommendations. Now for the rest...
Read MoreHow to Select the Right Tool Steel for Mold Cavities
With cavity steel or alloy selection there are many variables that can dictate the best option.
Read MoreHow to Get Rid of Bubbles in Injection Molding
First find out if they are the result of trapped gas or a vacuum void. Then follow these steps to get rid of them.
Read MoreRead Next
The Effects of Time on Polymers
Last month we briefly discussed the influence of temperature on the mechanical properties of polymers and reviewed some of the structural considerations that govern these effects.
Read MoreThe Effects of Temperature
The polymers we work with follow the same principles as the body: the hotter the environment becomes, the less performance we can expect.
Read MoreProcessor Turns to AI to Help Keep Machines Humming
At captive processor McConkey, a new generation of artificial intelligence models, highlighted by ChatGPT, is helping it wade through the shortage of skilled labor and keep its production lines churning out good parts.
Read More
.jpg;width=70;height=70;mode=crop)




















.png;maxWidth=300;quality=90)