PART ONE How to Determine Viscosity Data Using a Slit-Die Viscometer
Using a slit-die viscometer connected to a lab extruder can generate accurate data on melt viscosity at various shear rates and temperatures, a useful QC tool in processing.
Data on melt viscosity at various shear rates is useful in most extrusion operations. Such data provides a valuable quality-control tool. Viscosity data is required in screw and die design and also in computer simulation of the extrusion process. Proper selection of an extruder screw or extrusion die requires knowledge of viscosity as a function of shear rate. Further, instabilities such as melt fracture can be quantified and predicted if viscosity data is obtained from a capillary rheometer or slit-die viscometer.
This article describes how a slit-die viscometer connected to a laboratory extruder can be used to obtain accurate data on melt viscosity at various shear rates and temperatures. The slit-die viscometer provides an inexpensive method to determine rheological data, especially when compared with a capillary rheometer.
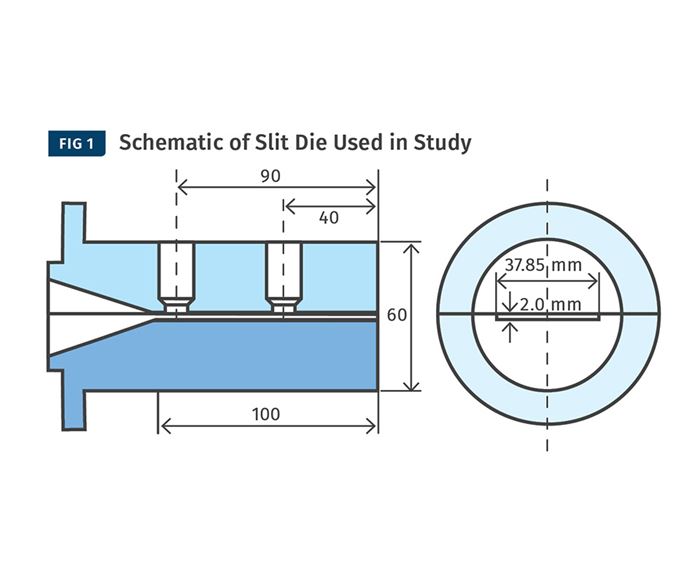
A slit die has a rectangular channel with a width W much greater than the height H (W>>H). The slit die can be connected directly to a laboratory extruder. Alternatively, a gear pump can be placed between the extruder and the slit die. Figure 1 shows a schematic drawing of the slit die used in this study.
The shear rate at the wall of the slit die is a function of the flow rate. The apparent shear rate can be determined from the following expression:
(apparent wall shear rate) = (flow rate) × (6/H2W)
The actual shear rate for a power-law fluid with power-law index n is:
(actual wall shear rate) = (apparent wall shear rate) × (0.667+0.333/n)
The shear stress at the wall can be determined from the pressure gradient of the measured pressure profile:
(shear stress) = 0.5H × (pressure gradient)
With the shear stress and the shear rate we can determine the shear viscosity:
(shear viscosity) = (shear stress) ÷ (shear rate)
In order to get viscosity data over a range of shear rate, the extruder has to run at various screw speeds. Each screw speed corresponds to a certain flow rate and thus to a certain shear rate. In order to determine the flow rate, the extruder output for each screw speed has to be measured. When a gear pump is used, the speed of the gear pump can be used to determine the flow rate and shear rate.
COLLECTING DATA & CALCULATING VISCOSITY
Data collection is easy when a data-acquisition system (DAS) is available. In this study the data were obtained on a 1-in. single-screw extruder at the Graham Engineering Corp. (GEC) lab in York, Pa. The extrusion runs with the slit die were part of a seminar workshop conducted by Rauwendaal Extrusion Engineering in cooperation with GEC. The data-acquisition software was part of the Navigator XC300 control system used by GEC.
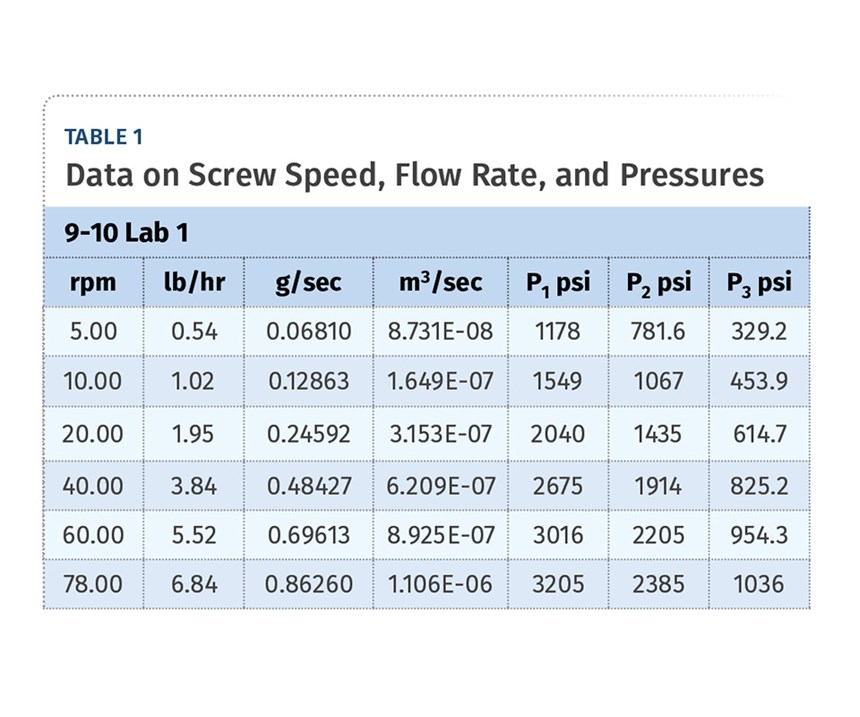
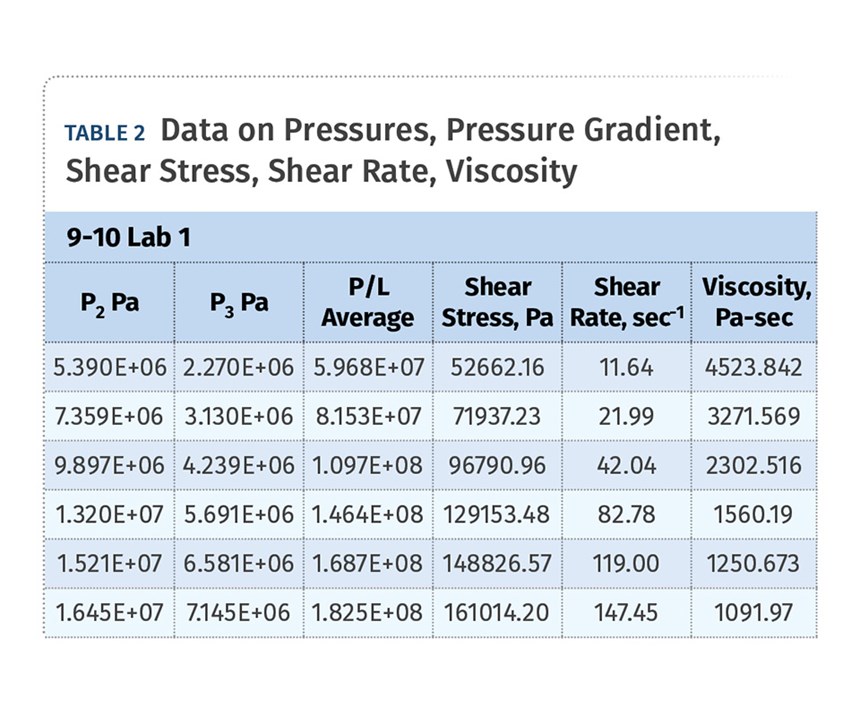
The DAS data was exported to Excel in a CSV (comma-separated values) format. Table 1 shows data on screw speed, flow rate, and pressures for lab 1 with extruder and die temperatures at 400 F. In Excel the data can be processed in a variety of ways. This allows automatic determination of the pressure gradient, shear stress, shear rate, and shear viscosity.
The polymer was a 0.5 MI HDPE 273-83 made by Kazanorgsintez, Kazan, Tatarstan, a republic of the Russian Federation. The extruder was run at six screw speeds: 5, 10, 20, 40, 60, and 78 rpm. The maximum screw speed on this extruder was 80 rpm. These screw speeds correspond to a shear-rate range from about 10 sec-1 to 150 sec-1. The extruder was run at two temperatures, 400 F (204.4 C) and 440 F (226.7 C).
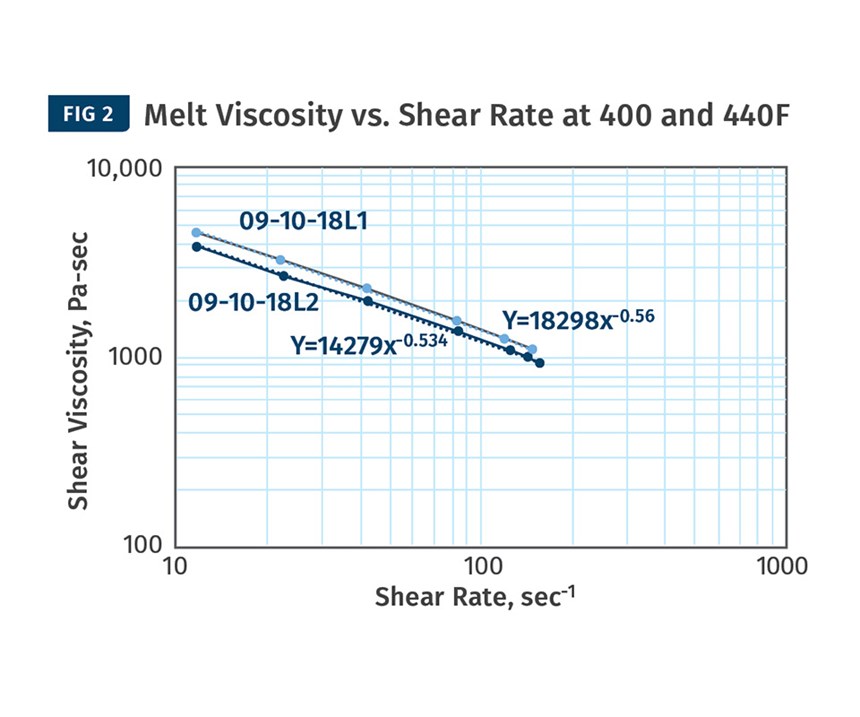
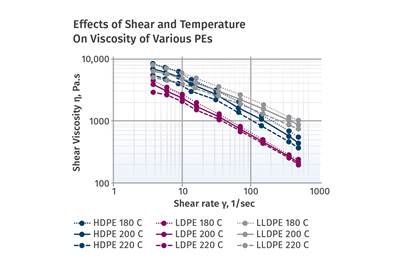
At this point we have enough information to determine the melt viscosity as a function of shear rate. Figure 2 shows the viscosity versus shear rate at two temperatures using a log-log plot. On a log-log plot the data fits well with a straight line. This means that the viscosity can be expressed as a function of shear rate using the power-law equation.
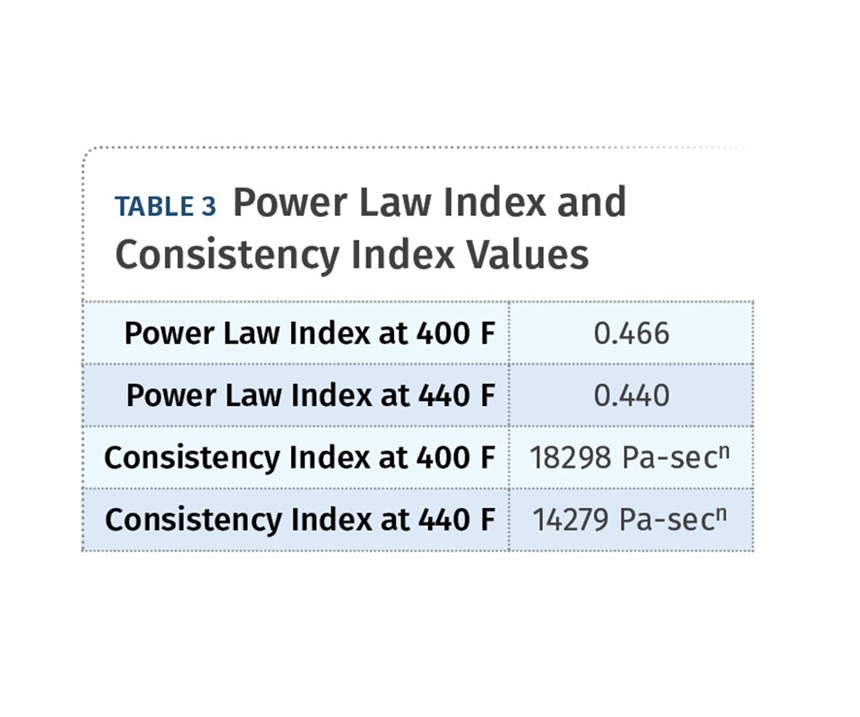
Figure 2 shows the power-curve equations. From these equations we can determine the consistency index and the power-law index at the two temperatures. The power-law parameters are shown in Table 3.
We see a small change (about 5%) in the value of the power-law index from 400 F to 440 F. The consistency index drops significantly (about 30%) from 400 F to 440 F.
We now have data on viscosity vs. shear rate at two temperatures. At this point it is useful to analyze some performance characteristics of the extruder. That will be the focus of Part 2 of this series.
ABOUT THE AUTHOR: Dr. Chris Rauwendaal is a well-known author, lecturer, researcher, entrepreneur, and consultant in the field of extrusion. He holds numerous patents and has written more than 200 articles and seven books related to extrusion, mixing, injection molding, and statistical process control. A Fellow of the Society of Plastics Engineers (SPE), he is the developer of the CRD, VIP, and ASM mixing technologies that utilize strong elongational flow to improve mixing in extrusion and molding. Rauwendaal also developed the HHT (high-heat-transfer) extruder screw designed to improve cooling in foam tandem and other extrusion operations. In 1990 he founded and is still president of Rauwendaal Extrusion Engineering. Contact: (530) 269-1082; chris@rauwendaal.com; rauwendaal.com.
Related Content
Green’s the Theme in Extrusion/Compounding
The drive toward circular economy is requiring processors to make more use of PCR. Machine builders at K—across all extrusion processes—will be highlighting innovations to help them do just that.
Read MorePregis Performance Flexibles: In the ‘Wow’ Business
Pregis went big and bold with investment in a brand-new, state-of-the-art plant and spent big on expanding an existing facility. High-tech lines, well-known leadership and a commitment to sustainability are bringing the “wow” factor to blown film.
Read MoreFlexible-Film Processor Optimizes All-PE Food Packaging
Tobe Packaging’s breakthrough was to create its Ecolefin PE multilayer film that could be applied with a specialized barrier coating.
Read MoreVariable-Diameter Air Ring Cools Film Above Frost Line
Tower-mounted adjustable ring adds cooling so that blown film can run at optimum line speeds with structures having soft inner skin layers that tend to block.
Read MoreRead Next
Understanding Viscosity in Extrusion
Both the power-law coefficient and the consistency index must be considered to calculate viscosity.
Read MorePart Two: Determining Viscosity Data Using a Slit Die Viscometer
Using a slit-die viscometer connected to a lab extruder can generate accurate data on melt viscosity at various shear rates and different temperatures—a useful QC tool in processing.
Read MoreUnderstanding the Effect of Polymer Viscosity on Melt Temperature
Every processor should get hold of the viscosity curves for the polymers they use or contemplate using in their operations, and learn how to read them.
Read More