How Much Horsepower Do You Need?
Most extrusion processors know that in order to boost output you need more power.
Most extrusion processors know that in order to boost output you need more power. But how are output and power connected? How do you know how much power you really need? And why is so much power required in the first place? Knowing the answers to these questions can help you spec a new machine, or troubleshoot an existing one.
Let’s answer the last question first. Extruders melt polymers primarily by viscous dissipation. Think of the amount of work it would take to rapidly stir a pot of heavy oil or grease with a spoon. That’s approximately what an extruder has to do to melt polymer.
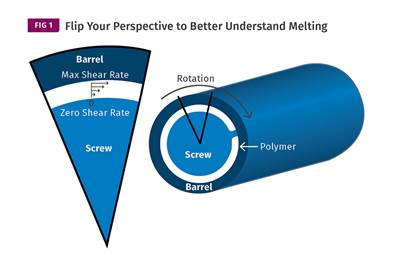
Almost all melting results from the rotation of the screw in the barrel. The turning screw causes the melt film adhering to the barrel to be stretched, or sheared. The extruder drive powers the screw rotation, overcoming the resistance required to stretch the melt film. The drive energy is thus transferred into the melt film (see the area in red in the diagram). This energy increases the film temperature and melts some of the adjacent unmelted material (shown in gray) by transferred heat.
The rotating screw flights continuously scrape the melt film off the barrel wall, collecting it on the front side of the flight. At the same time, the channels become shallower in the compression section of the screw, causing the unmelted resin to be pushed continuously toward the barrel wall. This renewal process allows for polymer to be melted faster without relying solely on conduction of heat from the melt film on the barrel wall.
Most melting occurs in the compression or barrier section of a single screw by means of this mechanism and accounts for approximately 85% to 90% of the drive power requirement. It is important to note that the same stretching or shearing mechanism occurs in the metering section. But there, the melt-film thickness equals the channel depth because most, if not all, the polymer is melted at that point.
So, how much power does it take to melt the polymer? That depends on the output rate, the specific heat of that particular polymer, and the final melt temperature. People versed in extrusion generally recognize that power requirements increase with output and melt temperature, but what role does the specific heat of the polymer play? The specific heat is a measure of the amount of energy required to raise 1 lb of polymer by 1° F. By taking the output and multiplying by the specific heat and the increase in temperature, you can estimate the energy (Btu/hr) required for melting. However converting this to drive horsepower requires taking into account all of the losses incurred in the system, such as drive efficiency, gearbox losses, heat lost to the environment or cooling system, and the power required for solids conveying and melt pressurization.
Experience shows that these losses normally add up to about 35% additional energy, so the calculated Btu/hr should be multiplied by 1.35 and a conversion factor (0.000393 hp/(Btu/hr): 0.000393 x 1.35= 0.00053.
Therefore: hp required = 0.00053 x (lb/hr) x (specific heat) x (temperature rise in the barrel).
For example, with 1000 lb/hr output and 470 F melt temperature processing HDPE:
HP = 0.00053 x (1000 lb/hr) x (0.55 Btu/hr-°F) x (470-70 F) = 116.6 hp.
(The temperature rise is the melt temperature minus the polymer feed temperature, with 70 F being the room temperature here.)
Keep in mind that this formula calculates the actual horsepower required and leaves no margin for drive overloads, so it is necessary to add the percentage of additional power you feel comfortable with. I add 15% to 20% for overloads, startups, etc. when calculating required drive power for a new extruder.
Calculation with this formula requires only the specific heat and simple multiplication. It can be used for sizing drives for new extruders or troubleshooting existing ones. For example, if the melt temperature or motor load is not known or the meter/indicator for either parameter is in question, the value of that parameter can be approximated with the formula. Try it and see if it works for you.
About the Author
Jim Frankland is a mechanical engineer who has been involved in all types of extrusion processing for more than 40 years. He is now president of Frankland Plastics Consulting, LLC. Contact jim.frankland@comcast.net or (724)651-9196.
Related Content
How to Estimate and Control Head Pressure
You rightfully worry about melt temperature, but don’t overlook head pressure, because the two are closely linked and will influence line performance.
Read MoreExtruder Alignment: Important, but Only Half the Equation
The other half? Aligning and supporting downstream equipment. Here are best practices.
Read MoreAvoid Four Common Traps In Granulation
Today, more than ever, granulation is an important step in the total production process. Our expert explains a few of the many common traps to avoid when thinking about granulators
Read MoreFully Automated Extrusion Process Enables Use of Composites for Manufacturing Pressure Tanks
Amtrol was looking for a more cost-effective means to produce thin-wall liners for a new line of pressure tanks. With the help of a team of suppliers, they built one of the world’s most sophisticated extrusion lines.
Read MoreRead Next
Understanding Melting in Single-Screw Extruders
You can better visualize the melting process by “flipping” the observation point so that the barrel appears to be turning clockwise around a stationary screw.
Read MorePeople 4.0 – How to Get Buy-In from Your Staff for Industry 4.0 Systems
Implementing a production monitoring system as the foundation of a ‘smart factory’ is about integrating people with new technology as much as it is about integrating machines and computers. Here are tips from a company that has gone through the process.
Read MoreHow Polymer Melts in Single-Screw Extruders
Understanding how polymer melts in a single-screw extruder could help you optimize your screw design to eliminate defect-causing solid polymer fragments.
Read More
.jpg;width=70;height=70;mode=crop)